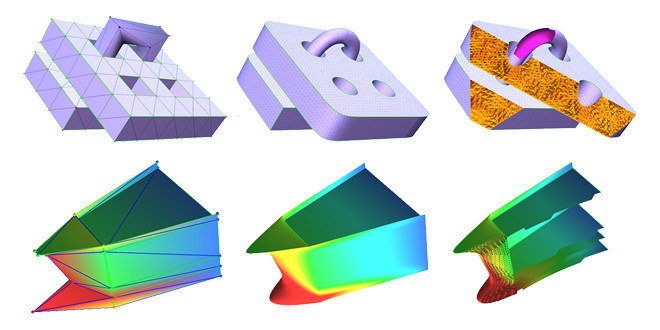
Top: A model of a mechanical part with the complex topology. Bottom: A material property representation with a ship model. Both are represented by our unified subdivision.

Yu-Sung Chang^† and Hong Qin^‡

^†Wolfram Research, Inc., 100 Trade Center Drive, Champaign, IL
^‡Department of Computer Science, State University of New York at Stony Brook

Computer-Aided Design, Vol. 38, No. 7, July 2006, pages 770-785

This paper presents a new unified subdivision scheme that is defined over a k-simplicial complex in n-D space with k<=3. We first present a series of definitions to facilitate topological inquiries during the subdivision process. The scheme is derived from the double (k+1)-directional box splines over k-simplicial domains. Thus, it guarantees a certain level of smoothness in the limit on a regular mesh. The subdivision rules are modified by spatial averaging to guarantee C¹ smoothness near extraordinary cases. Within a single framework, we combine the subdivision rules that can produce 1-, 2-, and 3-manifolds in arbitrary n-D space. Possible solutions for non-manifold regions between the manifolds with different dimensions are suggested as a form of selective subdivision rules according to user preference.We briefly describe the subdivision matrix analysis to ensure a reasonable smoothness across extraordinary topologies, and empirical results support our assumption. In addition, through modifications, we show that the scheme can easily represent objects with singularities, such as cusps, creases, or corners. We further develop local adaptive refinement rules that can achieve level-of-detail control for hierarchical modeling. Our implementation is based on the topological properties of a simplicial domain. Therefore, it is flexible and extendable. We also develop a solid modeling system founded on our subdivision schemes to show potential benefits of our work in industrial design, geometric processing, and other applications.

• Download: PDF